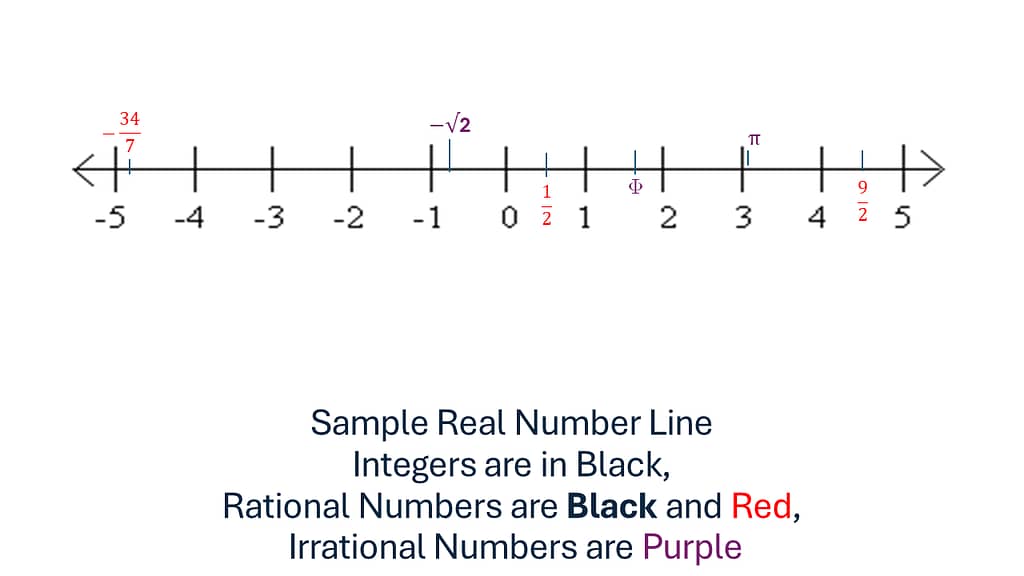
What is an Irrational Number?
An irrational number is a real number that cannot be expressed as a simple fraction of two integers. Unlike rational numbers, which can be written in the form \(\frac{a}{b}\) where a and b are integers and b is not equal to zero, irrational numbers cannot be represented by such a ratio.
Instead, they are characterized by non-repeating, non-terminating decimal expansions or as a radical number such as \(\sqrt{7}\)
Familiar examples of irrational numbers include:
- The square root of 2 \(\sqrt{2}\)
- Pi (π)
- The golden ratio (ϕ)
These numbers possess infinitely many decimal digits that neither terminate nor repeat in a pattern, making their precise representation impossible using fractions or finite decimal expressions.
Why Study Irrational Numbers?
- Completeness of the Real Number System: The inclusion of irrational numbers completes the real number system, which encompasses all possible values on the number line. By incorporating irrational numbers, mathematicians bridge the gap between rational numbers and continuous quantities, ensuring that no gaps or discontinuities exist in mathematical representations of real-world phenomena.
- Geometric and Algebraic Applications: Irrational numbers frequently arise in geometric constructions and algebraic equations. For instance, the square root of 2 appears in the context of right triangles with sides of equal length, while pi emerges in formulas for calculating the circumference and area of circles. The golden ratio exhibits remarkable properties in art, architecture, and aesthetics, influencing designs and proportions in various fields.
- Transcendental Functions: Many fundamental mathematical functions, such as the exponential function, and the trigonometric functions sine and cosine, involve irrational numbers. These transcendental functions play crucial roles in modeling natural phenomena, solving differential equations, and understanding the behavior of complex systems.
- Foundations of Number Theory: Irrational numbers contribute to the rich tapestry of number theory, a branch of mathematics that explores the properties and relationships of integers and their extensions. Studying irrational numbers deepens our understanding of number patterns, primes, and Diophantine equations, paving the way for groundbreaking discoveries and conjectures in pure mathematics.
Conclusion
In conclusion, irrational numbers represent an integral component of the mathematical landscape, imbued with profound significance. By embracing the complexity of irrational numbers, mathematicians unlock new realms of knowledge and illuminate the intricate symphony of patterns and structures that underlie the fabric of the universe.